If you need to raise a specific number to a power, you can use . Now we will take a closer look at properties of degrees.
Exponential numbers open up great possibilities, they allow us to transform multiplication into addition, and adding is much easier than multiplying.
For example, we need to multiply 16 by 64. The product of multiplying these two numbers is 1024. But 16 is 4x4, and 64 is 4x4x4. That is, 16 by 64 = 4x4x4x4x4, which is also equal to 1024.
The number 16 can also be represented as 2x2x2x2, and 64 as 2x2x2x2x2x2, and if we multiply, we again get 1024.
Now let's use the rule. 16=4 2, or 2 4, 64=4 3, or 2 6, at the same time 1024=6 4 =4 5, or 2 10.
Therefore, our problem can be written differently: 4 2 x4 3 =4 5 or 2 4 x2 6 =2 10, and each time we get 1024.
We can solve a number of similar examples and see that multiplying numbers with powers reduces to adding exponents, or exponential, of course, provided that the bases of the factors are equal.
Thus, without performing multiplication, we can immediately say that 2 4 x2 2 x2 14 = 2 20.
This rule is also valid when dividing numbers with powers, but in this case the exponent of the divisor is subtracted from the exponent of the dividend. Thus, 2 5:2 3 =2 2, which in ordinary numbers is equal to 32:8 = 4, that is, 2 2. Let's summarize:
a m x a n =a m+n, a m: a n =a m-n, where m and n are integers.
At first glance it may seem that this is multiplying and dividing numbers with powers not very convenient, because first you need to represent the number in exponential form. It is not difficult to represent the numbers 8 and 16, that is, 2 3 and 2 4, in this form, but how to do this with the numbers 7 and 17? Or what to do in cases where a number can be represented in exponential form, but the bases for exponential expressions of numbers are very different. For example, 8x9 is 2 3 x 3 2, in which case we cannot sum the exponents. Neither 2 5 nor 3 5 are the answer, nor does the answer lie in the interval between these two numbers.
Then is it worth bothering with this method at all? Definitely worth it. It provides enormous benefits, especially for complex and time-consuming calculations.
How to multiply powers? Which powers can be multiplied and which cannot? How to multiply a number by a power?
In algebra, you can find a product of powers in two cases:
1) if the degrees have the same bases;
2) if the degrees have the same indicators.
When multiplying powers with the same bases, the base must be left the same, and the exponents must be added:
When multiplying degrees with the same indicators, the overall indicator can be taken out of brackets:
Let's look at how to multiply powers using specific examples.
The unit is not written in the exponent, but when multiplying powers, they take into account:
When multiplying, there can be any number of powers. It should be remembered that you don’t have to write the multiplication sign before the letter:
In expressions, exponentiation is done first.
If you need to multiply a number by a power, you should first perform the exponentiation, and only then the multiplication:
www.algebraclass.ru
Addition, subtraction, multiplication, and division of powers
Addition and subtraction of powers
It is obvious that numbers with powers can be added like other quantities , by adding them one after another with their signs.
So, the sum of a 3 and b 2 is a 3 + b 2.
The sum of a 3 - b n and h 5 -d 4 is a 3 - b n + h 5 - d 4.
Odds equal powers of identical variables can be added or subtracted.
So, the sum of 2a 2 and 3a 2 is equal to 5a 2.
It is also obvious that if you take two squares a, or three squares a, or five squares a.
But degrees various variables And various degrees identical variables, must be composed by adding them with their signs.
So, the sum of a 2 and a 3 is the sum of a 2 + a 3.
It is obvious that the square of a, and the cube of a, is not equal to twice the square of a, but to twice the cube of a.
The sum of a 3 b n and 3a 5 b 6 is a 3 b n + 3a 5 b 6.
Subtraction powers are carried out in the same way as addition, except that the signs of the subtrahends must be changed accordingly.
Or:
2a 4 - (-6a 4) = 8a 4
3h 2 b 6 — 4h 2 b 6 = -h 2 b 6
5(a - h) 6 - 2(a - h) 6 = 3(a - h) 6
Multiplying powers
Numbers with powers can be multiplied, like other quantities, by writing them one after the other, with or without a multiplication sign between them.
Thus, the result of multiplying a 3 by b 2 is a 3 b 2 or aaabb.
Or:
x -3 ⋅ a m = a m x -3
3a 6 y 2 ⋅ (-2x) = -6a 6 xy 2
a 2 b 3 y 2 ⋅ a 3 b 2 y = a 2 b 3 y 2 a 3 b 2 y
The result in the last example can be ordered by adding identical variables.
The expression will take the form: a 5 b 5 y 3.
By comparing several numbers (variables) with powers, we can see that if any two of them are multiplied, then the result is a number (variable) with a power equal to amount degrees of terms.
So, a 2 .a 3 = aa.aaa = aaaaa = a 5 .
Here 5 is the power of the multiplication result, which is equal to 2 + 3, the sum of the powers of the terms.
So, a n .a m = a m+n .
For a n , a is taken as a factor as many times as the power of n;
And a m is taken as a factor as many times as the degree m is equal to;
That's why, powers with the same bases can be multiplied by adding the exponents of the powers.
So, a 2 .a 6 = a 2+6 = a 8 . And x 3 .x 2 .x = x 3+2+1 = x 6 .
Or:
4a n ⋅ 2a n = 8a 2n
b 2 y 3 ⋅ b 4 y = b 6 y 4
(b + h - y) n ⋅ (b + h - y) = (b + h - y) n+1
Multiply (x 3 + x 2 y + xy 2 + y 3) ⋅ (x - y).
Answer: x 4 - y 4.
Multiply (x 3 + x – 5) ⋅ (2x 3 + x + 1).
This rule is also true for numbers whose exponents are negative.
1. So, a -2 .a -3 = a -5 . This can be written as (1/aa).(1/aaa) = 1/aaaaa.
2. y -n .y -m = y -n-m .
3. a -n .a m = a m-n .
If a + b are multiplied by a - b, the result will be a 2 - b 2: that is
The result of multiplying the sum or difference of two numbers is equal to the sum or difference of their squares.
If the sum and difference of two numbers raised to square, the result will be equal to the sum or difference of these numbers in fourth degrees.
So, (a - y).(a + y) = a 2 - y 2.
(a 2 - y 2)⋅(a 2 + y 2) = a 4 - y 4.
(a 4 - y 4)⋅(a 4 + y 4) = a 8 - y 8.
Division of degrees
Numbers with powers can be divided like other numbers, by subtracting from the dividend, or by placing them in fraction form.
Thus, a 3 b 2 divided by b 2 is equal to a 3.
Writing a 5 divided by a 3 looks like $\frac $. But this is equal to a 2 . In a series of numbers
a +4 , a +3 , a +2 , a +1 , a 0 , a -1 , a -2 , a -3 , a -4 .
any number can be divided by another, and the exponent will be equal to difference indicators of divisible numbers.
When dividing degrees with the same base, their exponents are subtracted..
So, y 3:y 2 = y 3-2 = y 1. That is, $\frac = y$.
And a n+1:a = a n+1-1 = a n . That is, $\frac = a^n$.
Or:
y 2m: y m = y m
8a n+m: 4a m = 2a n
12(b + y) n: 3(b + y) 3 = 4(b +y) n-3
The rule is also true for numbers with negative values of degrees.
The result of dividing a -5 by a -3 is a -2.
Also, $\frac: \frac = \frac .\frac = \frac = \frac $.
h 2:h -1 = h 2+1 = h 3 or $h^2:\frac = h^2.\frac = h^3$
It is necessary to master multiplication and division of powers very well, since such operations are very widely used in algebra.
Examples of solving examples with fractions containing numbers with powers
1. Decrease the exponents by $\frac $ Answer: $\frac $.
2. Decrease exponents by $\frac$. Answer: $\frac$ or 2x.
3. Reduce the exponents a 2 /a 3 and a -3 /a -4 and bring to a common denominator.
a 2 .a -4 is a -2 the first numerator.
a 3 .a -3 is a 0 = 1, the second numerator.
a 3 .a -4 is a -1 , the common numerator.
After simplification: a -2 /a -1 and 1/a -1 .
4. Reduce the exponents 2a 4 /5a 3 and 2 /a 4 and bring to a common denominator.
Answer: 2a 3 /5a 7 and 5a 5 /5a 7 or 2a 3 /5a 2 and 5/5a 2.
5. Multiply (a 3 + b)/b 4 by (a - b)/3.
6. Multiply (a 5 + 1)/x 2 by (b 2 - 1)/(x + a).
7. Multiply b 4 /a -2 by h -3 /x and a n /y -3 .
8. Divide a 4 /y 3 by a 3 /y 2 . Answer: a/y.
Properties of degree
We remind you that in this lesson we will understand properties of degrees with natural indicators and zero. Powers with rational exponents and their properties will be discussed in lessons for 8th grade.
A power with a natural exponent has several important properties that allow us to simplify calculations in examples with powers.
Property No. 1
Product of powers
When multiplying powers with the same bases, the base remains unchanged, and the exponents of the powers are added.
a m · a n = a m + n, where “a” is any number, and “m”, “n” are any natural numbers.
This property of powers also applies to the product of three or more powers.
b b 2 b 3 b 4 b 5 = b 1 + 2 + 3 + 4 + 5 = b 15
6 15 36 = 6 15 6 2 = 6 15 6 2 = 6 17
(0.8) 3 · (0.8) 12 = (0.8) 3 + 12 = (0.8) 15
Please note that in the specified property we were talking only about the multiplication of powers with the same bases. It does not apply to their addition.
You cannot replace the sum (3 3 + 3 2) with 3 5. This is understandable if
count (3 3 + 3 2) = (27 + 9) = 36, and 3 5 = 243
Property No. 2
Partial degrees
When dividing powers with the same bases, the base remains unchanged, and the exponent of the divisor is subtracted from the exponent of the dividend.
(2b) 5: (2b) 3 = (2b) 5 − 3 = (2b) 2
11 3 − 2 4 2 − 1 = 11 4 = 44
Example. Solve the equation. We use the property of quotient powers.
3 8: t = 3 4
Answer: t = 3 4 = 81
Using properties No. 1 and No. 2, you can easily simplify expressions and perform calculations.
- Example. Simplify the expression.
4 5m + 6 4 m + 2: 4 4m + 3 = 4 5m + 6 + m + 2: 4 4m + 3 = 4 6m + 8 − 4m − 3 = 4 2m + 5
Example. Find the value of an expression using the properties of exponents.
2 11 − 5 = 2 6 = 64
Please note that in Property 2 we were only talking about dividing powers with the same bases.
You cannot replace the difference (4 3 −4 2) with 4 1. This is understandable if you calculate (4 3 −4 2) = (64 − 16) = 48, and 4 1 = 4
Property No. 3
Raising a degree to a power
When raising a degree to a power, the base of the degree remains unchanged, and the exponents are multiplied.
(a n) m = a n · m, where “a” is any number, and “m”, “n” are any natural numbers.

Please note that property No. 4, like other properties of degrees, is also applied in reverse order.
(a n · b n)= (a · b) n
That is, to multiply powers with the same exponents, you can multiply the bases, but leave the exponent unchanged.
2 4 5 4 = (2 5) 4 = 10 4 = 10,000
0.5 16 2 16 = (0.5 2) 16 = 1
In more complex examples, there may be cases where multiplication and division must be performed over powers with different bases and different exponents. In this case, we advise you to do the following.
For example, 4 5 3 2 = 4 3 4 2 3 2 = 4 3 (4 3) 2 = 64 12 2 = 64 144 = 9216
An example of raising a decimal to a power.
4 21 (−0.25) 20 = 4 4 20 (−0.25) 20 = 4 (4 (−0.25)) 20 = 4 (−1) 20 = 4 1 = 4
Properties 5
Power of a quotient (fraction)
To raise a quotient to a power, you can raise the dividend and the divisor separately to this power, and divide the first result by the second.
(a: b) n = a n: b n, where “a”, “b” are any rational numbers, b ≠ 0, n - any natural number.
(5: 3) 12 = 5 12: 3 12
We remind you that a quotient can be represented as a fraction. Therefore, we will dwell on the topic of raising a fraction to a power in more detail on the next page.
Powers and roots
Operations with powers and roots. Degree with negative ,
zero and fractional indicator. About expressions that have no meaning.
Operations with degrees.
1. When multiplying powers with the same base, their exponents are added:
a m · a n = a m + n .
2. When dividing degrees with the same base, their exponents are deducted .
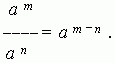
3. The degree of the product of two or more factors is equal to the product of the degrees of these factors.
4. The degree of a ratio (fraction) is equal to the ratio of the degrees of the dividend (numerator) and divisor (denominator):
(a/b) n = a n / b n .
5. When raising a power to a power, their exponents are multiplied:
All the above formulas are read and executed in both directions from left to right and vice versa.
EXAMPLE (2 3 5 / 15)² = 2² · 3² · 5² / 15² = 900 / 225 = 4 .
Operations with roots. In all the formulas below, the symbol means arithmetic root(the radical expression is positive).
1. The root of the product of several factors is equal to the product of the roots of these factors:
2. The root of a ratio is equal to the ratio of the roots of the dividend and the divisor:
![]()
3. When raising a root to a power, it is enough to raise to this power radical number:

4. If you increase the degree of the root by m times and at the same time raise the radical number to the mth power, then the value of the root will not change:
![]()
5. If you reduce the degree of the root by m times and simultaneously extract the mth root of the radical number, then the value of the root will not change:

Expanding the concept of degree. So far we have considered degrees only with natural exponents; but operations with powers and roots can also lead to negative, zero And fractional indicators. All these exponents require additional definition.
A degree with a negative exponent. The power of a certain number with a negative (integer) exponent is defined as one divided by the power of the same number with an exponent equal to the absolute value of the negative exponent:

Now the formula a m : a n = a m - n can be used not only for m, more than n, but also with m, less than n .
EXAMPLE a 4: a 7 = a 4 — 7 = a — 3 .
If we want the formula a m : a n = a m — n was fair when m = n, we need a definition of degree zero.
A degree with a zero index. The power of any non-zero number with exponent zero is 1.
EXAMPLES. 2 0 = 1, ( – 5) 0 = 1, (– 3 / 5) 0 = 1.
Degree with a fractional exponent. In order to raise a real number a to the power m / n, you need to extract the nth root of the mth power of this number a:
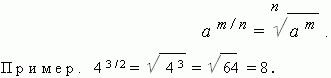
About expressions that have no meaning. There are several such expressions.
Where a ≠ 0 , does not exist.
In fact, if we assume that x is a certain number, then in accordance with the definition of the division operation we have: a = 0· x, i.e. a= 0, which contradicts the condition: a ≠ 0
— any number.
In fact, if we assume that this expression is equal to some number x, then according to the definition of the division operation we have: 0 = 0 · x. But this equality occurs when any number x, which was what needed to be proven.
0 0 — any number.
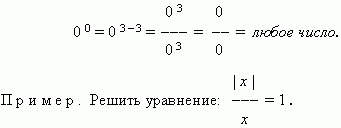
Solution. Let's consider three main cases:
1) x = 0 – this value does not satisfy this equation
2) when x> 0 we get: x/x= 1, i.e. 1 = 1, which means
What x– any number; but taking into account that in
in our case x> 0, the answer is x > 0 ;
Rules for multiplying powers with different bases
DEGREE WITH RATIONAL INDICATOR,
POWER FUNCTION IV
§ 69. Multiplication and division of powers with the same bases
Theorem 1. To multiply powers with the same bases, it is enough to add the exponents and leave the base the same, that is
Proof. By definition of degree
2 2 2 3 = 2 5 = 32; (-3) (-3) 3 = (-3) 4 = 81.
We looked at the product of two powers. In fact, the proven property is true for any number of powers with the same bases.
Theorem 2. To divide powers with the same bases, when the index of the dividend is greater than the index of the divisor, it is enough to subtract the index of the divisor from the index of the dividend, and leave the base the same, that is at t > p
(a =/= 0)
Proof. Recall that the quotient of dividing one number by another is the number that, when multiplied by the divisor, gives the dividend. Therefore, prove the formula where a =/= 0, it's the same as proving the formula
If t > p , then the number t - p will be natural; therefore, by Theorem 1
Theorem 2 is proven.
It should be noted that the formula
we have proved it only under the assumption that t > p . Therefore, from what has been proven, it is not yet possible to draw, for example, the following conclusions:
![]()
In addition, we have not yet considered degrees with negative exponents and we do not yet know what meaning can be given to expression 3 - 2 .
Theorem 3. To raise a degree to a power, it is enough to multiply the exponents, leaving the base of the degree the same, that is
Proof. Using the definition of degree and Theorem 1 of this section, we obtain:

Q.E.D.
For example, (2 3) 2 = 2 6 = 64;
![]()
518 (Oral) Determine X from the equations:
1) 2 2 2 2 3 2 4 2 5 2 6 = 2 x ; 3) 4 2 4 4 4 6 4 8 4 10 = 2 x ;
2) 3 3 3 3 5 3 7 3 9 = 3 x ; 4) 1 / 5 1 / 25 1 / 125 1 / 625 = 1 / 5 x .
519. (Set no.) Simplify:

520. (Set no.) Simplify:
521. Present these expressions in the form of degrees with the same bases:
1) 32 and 64; 3) 8 5 and 16 3; 5) 4 100 and 32 50;
2) -1000 and 100; 4) -27 and -243; 6) 81 75 8 200 and 3 600 4 150.
They have the same degrees, but the exponents of the degrees are not the same, 2² * 2³, then the result will be a base of the degree with the same identical base of the terms of the product of degrees, raised to an exponent equal to the sum of the exponents of all multiplied degrees.
2² * 2³ = 2²⁺³ = 2⁵ = 32
If the terms of a product of powers have different bases of powers, and the exponents are the same, for example, 2³ * 5³, then the result will be the product of the bases of these powers raised to an exponent equal to this same exponent.
2³ * 5³ = (2*5)³ = 10³ = 1000
If the powers being multiplied are equal to each other, for example, 5³ * 5³, then the result will be a power with a base equal to these identical bases of powers, raised to an exponent equal to the exponent of the powers, multiplied by the number of these identical powers.
5³ * 5³ = (5³)² = 5³*² = 5⁶ = 15625
Or another example with the same result:
5² * 5² * 5² = (5²)³ = 5²*³ = 5⁶ = 15625
Sources:
- What is a degree with a natural exponent?
- product of powers
Mathematical operations with powers can be performed only when the bases of the exponents are the same, and when there are multiplication or division signs between them. The base of an exponent is the number that is raised to a power.
Instructions
If the numbers are divisible by each other (cm 1), then y (in this example, this is the number 3) appears as a power, which is formed by subtracting the exponents. Moreover, this action is carried out directly: the second is subtracted from the first indicator. Example 1. Let us introduce: (a)b, where in brackets – a is the base, outside brackets – in – the exponent. (6)5: (6)3 = (6)5-3 = (6) 2 = 6*6 = 36. If the answer turns out to be a number to a negative power, then such a number is converted into an ordinary fraction, the numerator of which is one , and in the denominator the base with the exponent obtained from the difference, only in positive form (with a plus sign). Example 2. (2) 4: (2)6 = (2) 4-6 = (2) -2 = 1/(2)2 = ¼. The division of powers can be written in a different form, through the fraction sign, and not as indicated in this step through the “:” sign. This does not change the principle of the solution, everything is done exactly the same, only the entry will be made with a horizontal (or oblique) fraction sign instead of a colon. Example 3. (2) 4 / (2)6 = (2) 4-6 = (2 ) -2 = 1/(2)2 = ¼.
When multiplying identical bases that have degrees, the degrees are added. Example 4. (5) 2* (5)3 = (5)2+3 = (5)5 = 3125. If the exponents have different signs, then their addition is carried out according to mathematical laws. Example 5. (2)1* (2)-3 = (2) 1+(-3) = (2) -2 = 1/(2)2 = ¼.
If the bases of exponents differ, then most likely they can be brought to the same form by mathematical transformation. Example 6. Suppose we need to find the value of the expression: (4)2: (2)3. Knowing that the number four can be represented as two squared, this example is solved as follows: (4)2: (2)3 = (2*2)2: (2)3. Next, when raising a number to a power. Already having a degree, the degree indices are multiplied by each other: ((2)2)2: (2)3 = (2)4: (2)3 = (2) 4-3 = (2)1 = 2.
Helpful advice
Remember, if a given base seems different from the second base, look for a mathematical solution. Different numbers are not just given. Unless the typesetter made a typo in the textbook.
The power format of writing a number is a shortened form of writing the operation of multiplying a base by itself. With a number presented in this form, you can perform the same operations as with any other numbers, including raising them to a power. For example, you can raise the square of a number to an arbitrary power and obtaining the result at the current level of technological development will not pose any difficulty.

You will need
- Internet access or Windows calculator.
Instructions
To raise a square to a power, use the general rule for raising a square to a power that already has a power exponent. With this operation, the indicators are multiplied, but the base remains the same. If the base is denoted as x, and the initial and additional indicators as a and b, this rule can be written in general form as follows: (xᵃ)ᵇ=xᵃᵇ.
Operations with powers and roots. Degree with negative ,
zero and fractional indicator. About expressions that have no meaning.
Operations with degrees.
1. When multiplying powers with the same base, their exponents add up:
a m · a n = a m + n .
2. When dividing degrees with the same base, their exponents are deducted .
3. The degree of the product of two or more factors is equal to the product of the degrees of these factors.
(abc… ) n = a n· b n · c n…
4. The degree of a ratio (fraction) is equal to the ratio of the degrees of the dividend (numerator) and divisor (denominator):
(a/b ) n = a n / b n .
5. When raising a power to a power, their exponents are multiplied:
(a m ) n = a m n .
All the above formulas are read and executed in both directions from left to right and vice versa.
EXAMPLE (2 · 3 · 5 / 15)² = 2² 3² 5² / 15² = 900 / 225 = 4 .
Operations with roots. In all the formulas below, the symbol means arithmetic root(the radical expression is positive).
1. The root of the product of several factors is equal to the product roots of these factors:
2. The root of a ratio is equal to the ratio of the roots of the dividend and the divisor:
![]()
3. When raising a root to a power, it is enough to raise to this power radical number:
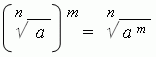
4. If we increase the degree of the root in m raise to m the th power is a radical number, then the value of the root will not change:
5. If we reduce the degree of the root in m extract the root once and at the same time m th power of a radical number, then the value of the root is not will change:
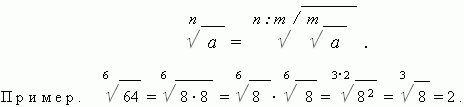
Expanding the concept of degree. So far we have considered degrees only with natural exponents; but actions with degrees and roots can also lead to negative, zero And fractional indicators. All these exponents require additional definition.
A degree with a negative exponent. Power of some number c a negative (integer) exponent is defined as one divided by a power of the same number with an exponent equal to the absolute valuenegative indicator:

T now the formula a m: a n= a m - n can be used not only form, more than n, but also with m, less than n .
EXAMPLE a 4 :a 7 = a 4 - 7 = a - 3 .
If we want the formulaa m : a n= a m - nwas fair whenm = n, we need a definition of degree zero.
A degree with a zero index. The power of any non-zero number with exponent zero is 1.
EXAMPLES. 2 0 = 1, ( – 5) 0 = 1, (– 3 / 5) 0 = 1.
Degree with a fractional exponent. To raise a real number and to the power m/n , you need to extract the root nth power of m -th power of this number A :
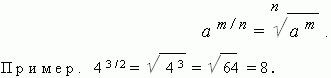
About expressions that have no meaning. There are several such expressions. any number.
In fact, if we assume that this expression is equal to some number x, then according to the definition of the division operation we have: 0 = 0 · x. But this equality occurs when any number x, which was what needed to be proven.
Case 3.
0 0 - any number.
Really,

Solution. Let's consider three main cases:
1) x = 0 – this value does not satisfy this equation
(Why?).
2) when x> 0 we get: x/x = 1, i.e. 1 = 1, which means
What x– any number; but taking into account that in
In our case x> 0, the answer isx > 0 ;
3) when x < 0 получаем: – x/x= 1, i.e. e . –1 = 1, therefore,
In this case there is no solution.
Thus, x > 0.
If two powers are multiplied (or divided), which have different bases, but the same exponents, then their bases can be multiplied (or divided), and the exponent of the result can be left the same as that of the factors (or dividend and divisor).
In general, in mathematical language, these rules are written as follows:
a m × b m = (ab) m
a m ÷ b m = (a/b) m
When dividing, b cannot be equal to 0, that is, the second rule must be supplemented with the condition b ≠ 0.
Examples:
2 3 × 3 3 = (2 × 3) 3 = 63 = 36 × 6 = 180 + 36 = 216
6 5 ÷ 3 5 = (6 ÷ 3) 5 = 2 5 = 32
Now, using these specific examples, we will prove that the rules-properties of degrees with the same exponents are correct. Let's solve these examples as if we don't know about the properties of degrees:
2 3 × 3 3 = (2 × 2 × 2) × (3 × 3 × 3) = 2 × 2 × 2 × 3 × 3 × 3 = 8 × 27 = 160 + 56 = 216
65 ÷ 35 = (6 × 6 × 6 × 6 × 6) ÷ (3 × 3 × 3 × 3 × 3) == 2 × 2 × 2 × 2 × 2 = 32
As we can see, the answers coincided with those obtained when the rules were used. Knowing these rules allows you to simplify calculations.
Note that the expression 2 × 2 × 2 × 3 × 3 × 3 can be written as follows:
(2 × 3) × (2 × 3) × (2 × 3).
This expression in turn is something other than (2 × 3) 3. that is, 6 3.
The considered properties of degrees with the same indicators can be used in the opposite direction. For example, what is 18 2?
18 2 = (3 × 3 × 2) 2 = 3 2 × 3 2 × 2 2 = 9 × 9 × 4 = 81 × 4 = 320 + 4 = 324
Properties of powers are also used when solving examples:
= 2 4 × 3 6 = 2 4 × 3 4 × 3 × 3 = 6 4 × 3 2 = 6 2 × 6 2 × 3 2 = (6 × 6 × 3) 2 = 108 2 = 108 × 108 = 108 ( 100 + 8) = 10800 + 864 = 11664



